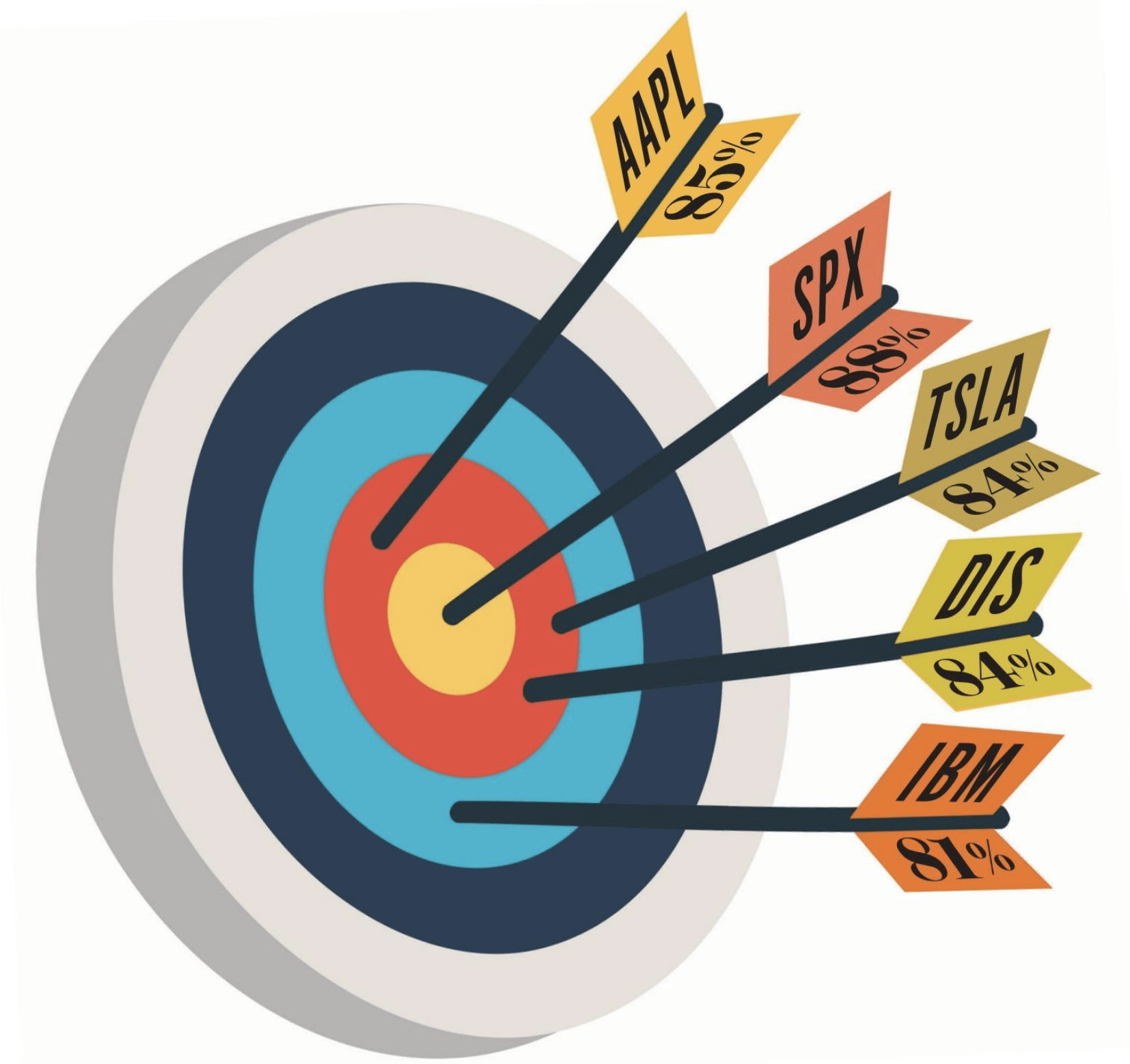
Probabilities Favor Higher Tuition
Sure, there’s a possibility the price of college might come down, but the high growth rate and low volatility say the odds are against it
If Ben Franklin were alive today, he might revise his maxim about death and taxes to include the rising cost of college. It’s uncertain which one people dread the most.
Tuition and fees plus room and board for all institutions have increased by 45% (accounting for continuous log growth) in the 20 years between 1999 and 2019, according to the National Center for Education Statistics. In comparison, median household income grew by 14.5% during that time, says the St. Louis Federal Reserve Bank. Ouch.
The August Luckbox Magazine will reveal new research that raises doubt on the economic value of thousands of majors and hundreds of universities. A recent study will be profiled that shows about one-third of 30,000 combinations of majors at 1700 different colleges and universities have a net negative return on investment. For those majors, rising tuition costs, college textbooks, housing, student debt expense and the opportunity cost of being out of the job market never catch up to the total investment.
If you are not a subscriber, you can sign up for free today to receive the Issue with Education in your inbox.
In the interim, consider that the discrepancy between income and college costs may have parents of college-age students and students themselves wondering what the probability is that tuition might come back down. After all, things can’t go up forever, can they? To answer that, turn to the probability models used for stocks.
The core of the model takes the potential percentage price change of a stock, for example, and converts it into a standard normal variable. That variable can be set into a normal distribution, from which the probability numbers are derived.
Now, the model for stocks assumes that we live in a risk-neutral world. In a risk-neutral world, a risky asset like a stock should be expected to grow by at least the risk-free rate in order to justify an investment in it.
In other words, why take the risk of buying a stock if it can’t earn at least as much as a risk-free T-bill? That’s why the model has the return of the stock increase by the risk-free rate, and a positive interest rate increases the probability of higher stock returns.
Offsetting the positive impact of interest rates is volatility, which, in the model, is subtracted from the interest rate. Without getting into the details of the volatility component of the formula, suffice it to say that volatility—any volatility—pulls stock returns down.
For example, a 1% increase on a $100 stock takes it to $101. If that’s followed by a 1% drop on the stock, it takes it down $1.01 to $99.99. Even though the +1% and -1% add to 0%, the stock’s price is still lower. That’s how volatility reduces the potential returns of stocks.
There’s a battle between interest rates and volatility over which affects probability more. Most of the time, and certainly for the past couple of decades in the United States, volatility in stocks has been higher than the risk-free rate. That theoretically increases the probability of lower stock prices. But when the risk-free rate is higher than volatility, the probability of higher prices increases.
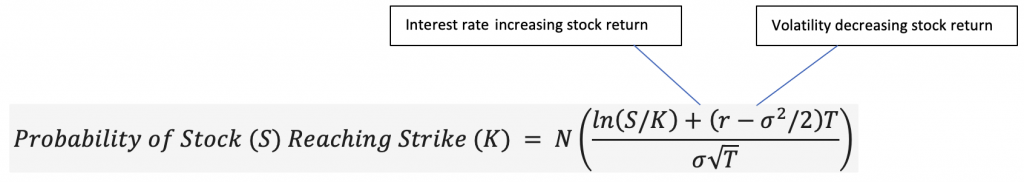
For example, if volatility is 25%, and the interest rate is 1.5%, there’s a 16% probability that a $100 stock will be above $126 in a year and a 16% probability it will be below $77. But if volatility is 25% and the interest rate is 10%, there’s a 16% probability that a $100 stock will be above $137 in a year and a 16% probability that it will be below $84. The higher interest rate shifts the probabilities to higher numbers. Smaller drops and larger increases are more likely.
That, unfortunately, is the situation with college tuition. The implied growth rate is high, and the volatility is relatively low. Over the past 20 years, the cost of college—tuition, room and board—has gone up by 2.3% per year, on average. The volatility of that annual increase is 1.2%—a little over half of the rate of increase.
That’s a case where the rate component is dominating the volatility component in the probability model and biasing the probability to higher prices. Even though college costs haven’t had a downtick in 20 years, the fact that in some years the increase is bigger and in other years less means there’s volatility. It’s just that the volatility isn’t very high.
So, the probabilities are just theoretical calculations and aren’t guarantees for either stock prices or college costs. But while there’s a possibility the price of college might come down, the high growth rate and low volatility say the odds are against it. Sorry.